
TwinDamp™: Improving the Damping Capacity of Manganese-Copper
Measurements comparing the damping capacity of our TwinDamp™ alloy to manganese-copper and stainless steel.

Transmission Path Evasion
An overview of the concept of vibration transmission path evasion and its incorporation into the design of the Carbide Base Diamond footer.

Survey of Audio Footer Designs
A survey of several audio equipment footers designs. The vibration isolation performance of each design is measured and graphed for relative comparison.
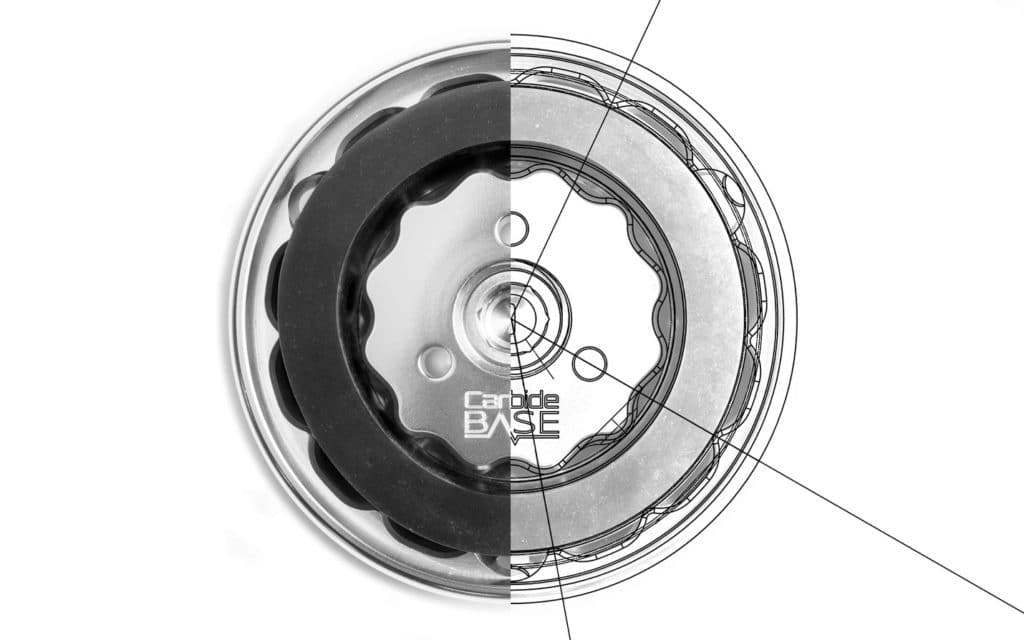
Utilizing Low Shape Factor Elastomers
An analysis of the novel design of the Carbide Base audio footer. The benefits of the low shape factor elastomers and unique ball bearing configuration utilized in the footer are quantified with measurements.

Vibration Dissipation Using Carbide Base Footers
Measurements comparing the vibration dissipation ability of Carbide Base footers to standard floor spikes. A test loudspeaker is constructed to analyze the damping effect that the footers have on cabinet panel resonances.

